Pythagorean theorem

Pythagorean theorem: in a right triangle, the sum of the squares of the catetos is equal to the square of the hypotenuse

James A. Garfield

Garfield was the twentieth president of the United States, was an amateur mathematician and published this demonstration in the magazine The New England Journal of Education (vol. 3, p. 161) in 1876, five years before his arrival at the White House and his death, as he died in September 1881, the year of his appointment, as a result of injuries sustained in an attack in July of that year

Demonstration: Pythagorean Theorem

Based on the following scheme:

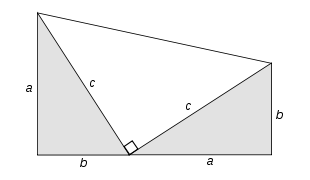
the demonstration is based on the observation that:

\text{area}(T_1) + \text{area}(T_2) + \text{area}(T_3) = \text{area}(T_1 \cup T_2 \cup T_3)

where T_1 is the triangle on the left, T_2 the triangle on the right and T_3 the central triangle

so we have to:

\text{area}(T_1)=\text{area}(T_2)=\frac{1}{2}\cdot a \cdot b
\text{area}(T_3)=\frac{1}{2}\cdot c^2

as T_1 \cup T_2 \cup T_3 is a trapezoid of bases a and b and height a + b you have to:

\text{area}(T_1 \cup T_2 \cup T_3)=\frac{1}{2} \cdot (a + b)^2

therefore if we replace in the initial formula we have:

2\cdot \frac{1}{2} \cdot a \cdot b + \frac{1}{2} \cdot c^2 = \frac{1}{2} \cdot (a + b)^2
2\cdot \frac{1}{2} \cdot a \cdot b + \frac{1}{2} \cdot c^2 = \frac{1}{2} \cdot (a^2 + a \cdot b + a \cdot b + b^2)
2\cdot \frac{1}{2} \cdot a \cdot b + \frac{1}{2} \cdot c^2 = \frac{1}{2} \cdot (a^2 + 2 \cdot a \cdot b + b^2)
2\cdot \frac{1}{2} \cdot a \cdot b + \frac{1}{2} \cdot c^2 = \frac{1}{2} \cdot (a^2 + b^2) + \frac{1}{2} \cdot 2 \cdot a \cdot b
\frac{1}{2} \cdot c^2 = \frac{1}{2} \cdot (a^2 + b^2) + \frac{1}{2} \cdot 2 \cdot a \cdot b - 2\cdot \frac{1}{2} \cdot a \cdot b
\frac{1}{2} \cdot c^2 = \frac{1}{2} \cdot (a^2 + b^2)
2 \cdot \frac{1}{2} \cdot c^2 = 2 \cdot \frac{1}{2} \cdot (a^2 + b^2)
c^2 =a^2 + b^2

with what the demonstration is concluded